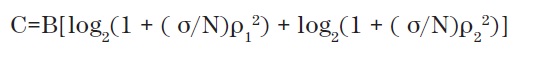The MIMO channel Matrix
Consider for a moment in time, on the same frequency and channel modeling as a black box with durable internal components. If we add two completely different input signals is to be mixed with each other in a certain manner depending on the value of Z1 to Z4. If we send a signal training that is unique for each input and output measurement, we know how it was connected, so as to disconnect them.
All signals, data, and training will be combined in the same way, so that’s what we learned from the training signal can be applied to real data. Noise and distortion of the modulation limit, which can be used along with the ability to uncouple the outputs. The worst case would be if Z1 to Z4 are the same, when both outputs are the same and MIMO does not operate.
The best case is if the outputs are equal in magnitude and opposite in phase, when theoretically double the capacity in The MIMO channel Matrix.
Equation 1 The long-form version of the theorem of channel capacity can be written as shown in the following figure
Where,
- C = channel capacity in bits per second,
- B = occupied bandwidth in Hz, σ/N = signal to noise ratio and
- ρ = a singular value of the channel matrix
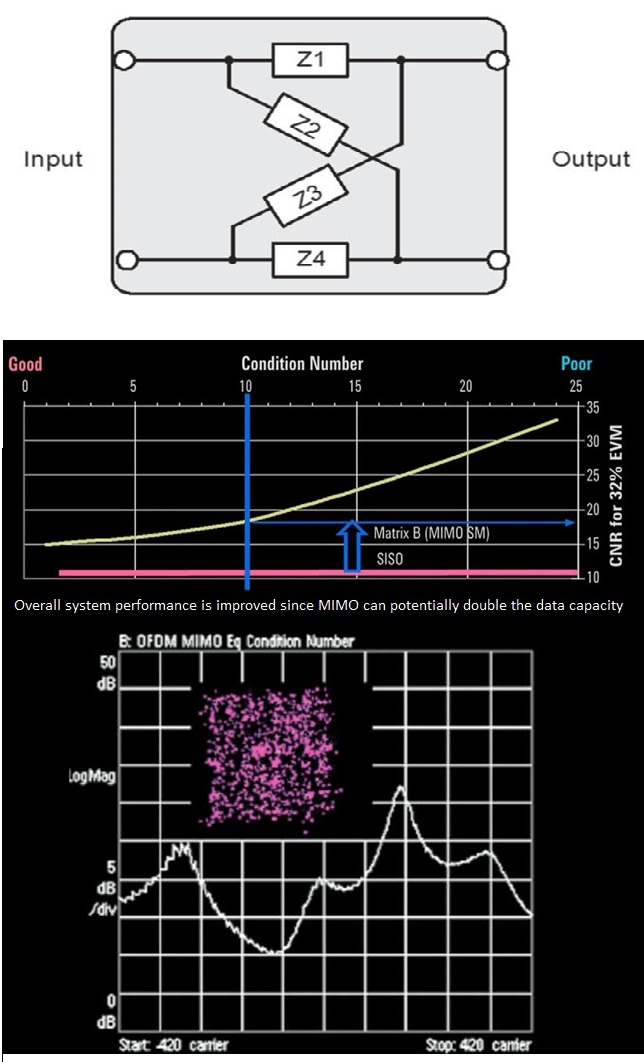
The potential in the instant system capacity increase can be derived from the relationship of singular values of the channel matrix H, also known as the condition number. The condition number of The MIMO channel Matrix can also be used to indicate the increase in SNR required recovering the MIMO signal in relation to the SISO case.
With the channel changing due to fading and trajectories multiple effects and Doppler frequency shift due to motion of phone, among others, the number of conditions to changes of constant frequency in the spectrum of RF channel, as is illustrated in the figure below.
Reference signals (or pilots) in places regular frequency at the output of each transmitter provides a way for recipients to estimate the channel coefficients. In general, each “tube” of data will not have the same performance. LTE uses feedback mechanisms known as pre-coding and make Eigen training – both forms of “closed-loop MIMO,” in the phone to request modifications to the cross-coupling of the transmitter outputs to give the best match to the MIMO channel Matrix in channel characteristics.